The Cognitive Origins of Human Abstract Geometry

By Moira R. Dillon

Philosophers throughout history have debated the relation between the abstract geometry in our unique human minds and the physical geometry in the world that humans share with other animals. Unlike other creatures, humans can conceive of abstract spatial entities—like infinitely small points and never-ending lines—regardless of culture or formal education [6]. These concepts help students learn formal geometry, such as Euclidean geometry. As with other animals, humans also intuitively interact with geometry in everyday life to navigate places and recognize objects. Are uniquely human abstract geometric concepts—like infinitesimal points and lines—rooted in evolutionarily ancient, everyday, and spatially finite experiences of places and objects? What are the cognitive origins of abstract geometry?

Two proposals address this question by appealing to the everyday spatial experiences that we share with other animals. One idea—based on decades of research in the psychological, cognitive, and neural sciences—suggests that the mental representations that support our everyday interactions with places and objects are not as unitary as they may seem. For example, seminal work on the search behavior of disoriented animals—from fish and chickens to rats and humans—has found that when humans and other animals navigate simple rectangular environments, they tend to keep track of only the distances and directions of the environment’s boundaries [1, 5] (see Figure 1). In contrast, when they recognize objects, how far away an object is or what direction it is facing is less important than whether it has the right shape [9]. Facilitated by the combinatorial capacities of uniquely human symbol systems like language and pictures, the complementary geometries of these two foundational systems for navigation and object recognition may merge in every human’s development to support an intuitive natural geometry that combines distance, direction, and shape. This natural geometry allows us to comprehend abstract geometry and learn Euclidean geometry [10].

Figure 1. Young children who are disoriented in a rectangular environment use the environment’s distance and directional information to regain their heading. 1a. A young child is shown a sticker that is hidden under a disk at one of the rectangle’s corners (indicated by the blue arrow). The child is then blindfolded and slowly spun in the center of the environment to disorient them. When the blindfold is removed, the disoriented child is asked to find the sticker. The child is likely to search either in the correct corner of the rectangular environment or the geometrically equivalent, diagonally opposite corner. 1b. Children intuitively go to the left of a far wall. Non-human animals also exhibit this same use of distance and directional information to regain their heading. Figure courtesy of [7].

A related proposal for the cognitive origins of abstract geometry suggests that noisy, dynamic mental simulations of navigation—akin to a correlated random walk of a navigating insect—approximate Euclidean principles and ground abstract geometric reasoning. A series of behavioral experiments with children and adults suggest that by late childhood, humans can visualize planar figures like triangles and reason about these figures’ Euclidean properties (e.g., the fact that a triangle’s angle sizes sum to a constant) using dynamic mental simulations that can be modeled as a correlated random walk [3] (see Figure 2). For example, when children and adults are shown the bottom two corners of a series of fragmented planar triangles, they can intuitively estimate the locations of the triangles’ missing third corners. The slope of the log of the standard deviation of these estimates can then be calculated as a function of the log of triangle side length. This slope, or scaling exponent, is equivalent to the power law by which the standard deviation of localization estimates scales with triangle side length. The scaling exponent represents the global correction of the local noise that is associated with maintaining smooth motion in the direction of the given angle sizes:

\[d^2\theta/dt^2=1/\tau(1/\xi(\theta-\theta_0)-d\theta/dt)+\eta(t)\tag1\]

\[dx/dt=v_p\cos(\theta)\tag2\]

\[dy/dt=v_p\sin(\theta).\tag3\]

Figure 2. A correlated random walk model describes how older children and adults visualize planar figures like triangles and reason about their Euclidean properties. In this model, the local angle evolves with accompanying noise as the triangle’s side is extrapolated. The model parameters are as follows: an inertial relaxation timescale \(\tau\) for local smoothness; a characteristic speed \(v_p\) with which the coordinates progress; a timescale \(\xi\) for global error correction (illustrated as the number of segments between error-correction events); and a noise term \(\eta(t)\) with noise amplitude \(D\langle\eta(t)\eta(t^\prime)\rangle=\) \(D\delta(t-t^\prime)\) (not shown in the figure). The stopping threshold is denoted by \(\varepsilon\), and the base angle is denoted by \(\theta_0\). The right and left extrapolations are simulated independently and are not necessarily symmetrical. Figure adapted from [3].

Our work supports elements of both of these proposals. In a recent experiment, we investigated whether educated adults’ representations of abstract points and lines reflect the foundational geometry that humans and other animals use for navigation and object recognition (see Figure 3). Consistent with the first proposal, we found that representations of distance and direction for navigation—as well as shape for object recognition—are still present and active in the minds of human adults; in fact, they are even individually accessible through simple and minimally contrastive language that describes the very same simple planar figures [8]. And consistent with the second proposal, we discovered that a verbal description of a navigating agent uniquely elicits the same geometric information (e.g., distance and direction for navigation) as a verbal description of abstract points and lines. This latter finding suggests an intimate link between uniquely human abstract geometric intuitions and the specific geometric intuitions that we and other animals call upon in navigational contexts; we wander the abstract Euclidean plane like we wander the physical world [8].

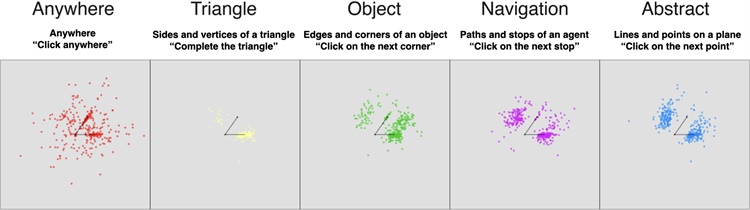
Figure 3. In a recent experiment, educated adults watched short videos in which two points and two line segments formed an open figure on an otherwise blank screen. Descriptions of these simple visuals with sparse and minimally contrasting language created different spatial contexts. After watching each video, participants had to click on the screen as indicated by the following prompts: anywhere (anywhere condition); to complete the triangle (triangle condition); on the next corner of the object (object condition); on the next stop on the agent’s path (navigation condition); or on the next point on the abstract plane (abstract condition). Participants produced strikingly different sets of geometric representations across spatial contexts. In particular, they tended to preserve distance and direction for open paths in the navigation condition but maintain length and angle for closed shapes in the object condition. The elicited geometry was nevertheless remarkably similar across the navigation and abstract conditions. Figure adapted from [8].

The relation of our recent findings to theories about the origins of human abstract geometry has implications for the development of geometry pedagogy and other educational approaches. The use of navigational frameworks to teach abstract geometry might better serve mathematics instruction [2], but lessons should also account for the ways in which an individual’s navigational experiences may affect their capacity for learning. Future experiments could examine the specific properties of navigation that might support the acquisition of foundational Euclidean concepts of linearity, parallelism, perpendicularity, and symmetry. If our explorations of abstract geometry often rely on spatial navigation, then the worlds in which we live and think coincide in ways that we can ultimately enhance to encourage more students to investigate the domains of geometry.

Moira R. Dillon is an assistant professor of psychology at New York University and director of the Lab for the Developing Mind. She uses cognitive, developmental, and computational approaches to gain insight into the origin and development of uniquely human cognition — from the basic sensitivities of infants to children’s untutored use of symbols and language to the high-level concepts of adults.