By Robert Saye and James Sethian
Foams are everywhere—in the head on a beer, in the soapsuds in a kitchen sink . . . . Liquid foams like these are also key ingredients in industrial manufacturing, where they are used in fire retardants, in froth floatation for separating substances, even in baking bread. Solidification of liquid foams can result in solid foams, which have remarkably strong compressible strength because of their pore-like internal structure; a common use of solid foams is in lightweight bicycle helmets.
Devising mathematical and numerical models for evolving foams is daunting. In a foam, thin fluid-containing membranes, called “lamellae,” separate pockets of air. As the foam evolves, fluid from the membranes drains into connecting rivers known as “Plateau borders,” until one of the membranes ruptures, disturbing the balance of forces between surface tension in the membranes and the trapped air pockets. In the ensuing rearrangement of the bubbles, macroscopic effects of gas dynamics compete with microscopic effects driven by fluid flow and surface tension, until a new equilibrium configuration is reached. All this is accompanied by continuing drainage, until another membrane ruptures and the cycle repeats itself.
What makes the modeling of foams so challenging is the vast range of space and time scales involved [6]. Consider an open, half-empty bottle of beer: It may seem that nothing is happening in the collection of interconnected bubbles near the top, but close examination of the currents in the lamellae separating the air pockets shows slow but steady drainage. With respect to time scales alone, it can take tens to hundreds of seconds for the fluid in a thin lamella to drain. Rupture of a membrane triggers an explosion at hundreds of centimeters a second, after which the imbalanced configuration rights itself to a new stable structure in less than a second. On the spatial side, the membranes are barely micrometers thick, while the large gas pockets can span many centimeters. All told, the biggest and smallest scales differ by roughly six orders of magnitude in both space and time.
Finding a static equilibrium configuration of bubbles, even without considering the fluid mechanics, has been a rich source of mathematical problems for centuries, drawing on aspects of minimal surfaces theory, optimization, partial differential equations, and differential geometry. One of the most powerful tools for computing energy-minimizing configurations in the absence of fluid flow has been Ken Brakke’s “Surface Evolver” [1], which constantly readjusts a discretized triangulation of the interface to lower the energy and converge to an optimal structure.
A recent article in Science magazine [3] described a mathematical approach developed by Berkeley mathematicians Robert Saye and James Sethian to tackle the combined multiscale effects of large-scale fluid and gas flow, drainage of lamellae, and membrane rupture, all within one computational model. The article caught the attention* of the popular press. According to the accompanying Science commentary [5], “This new class of numerical modelers has laid foundations for a fresh start.”
Saye and Sethian use a scale-separated approach to split the problem into three distinct phases that cycle continuously. During the rearrangement phase, a cluster of bubbles readjusts itself as surface tension in the membranes pushes on air in the pockets, according to equations of multi-phase incompressible flow, until a new macroscopic equilibrium is attained. Once this large-scale equilibrium is reached, the model invokes a drainage stage, in which liquid in the lamellae drains into the Plateau borders, obeying thin film equations. Once a membrane becomes too thin, the model removes it, kicking the cluster far from equilibrium and leading back to the rearrangement stage. As the model slides through these phases, the resulting foam cluster pops, readjusts, and drains in a continual bubble cascade.
At the heart of this computational model is a collection of new algorithmic techniques for solving the underlying equations of motion; Sethian presented this work in an invited talk (“Tracking Multiphase Physics: Geometry, Foams, and Thin Films”) at SIAM’s 2013 conference on materials science. For the rearrangement phase of their model, Saye and Sethian use their Voronoi implicit interface method (VIIM) [2,4] to capture multiphase flow. For the drainage step, the researchers have constructed an elaborate model that couples thin film equations for each individual lamella to similar equations for each of the connecting Plateau borders; the coupled system is then solved in a finite element scheme on a triangulated mesh of the set of curved interconnected surfaces and junctions. When a lamella is thin enough, the model removes it, causing the gas pockets on each side to merge. This moves the system out of macroscopic balance, and the rearrangement module is then re-invoked.
As an added bonus, by using the membrane thickness as input to well-known methods for solving the thin film interference equations, Saye and Sethian determined how light is reflected from the evolving structures. The resulting images (Figure 1), which appeared in their Science article, show the dynamics of a foam cluster as it evolves through the multiscale sequence of rearrangement, drainage, and rupture, visualized through computation of the thin film patterns generated by a beach scene reflected from the evolving cluster.
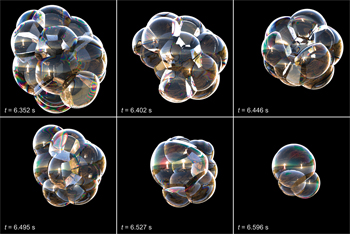
Figure 1. Reflections on an evolving bubble cluster, combining macroscopic rearrangement, drainage of lamellae, and rupture [3].
|
The model, while powerful, is missing some important effects. “To get a more complete picture,” Sethian says, “we need to include additional physical effects, such as diffusive coarsening and different types of surface rheologies, including liquid–gas interfaces with more elaborate surface viscosities, evaporation dynamics, and heating.”
Looking ahead, he and Saye plan to use this approach to study industrial applications and problems related to multiphase multiphysics. Their current interests include biological cell cluster modeling and industrial foams.
*And the fancy—articles that appeared shortly afterward bore such titles as “Heady Mathematics,” “The Life Cycle of a Bubble,” “The Secret Lives of Bubbles,” “Pop the Champagne: Bubble Mystery Solved,” and even “Physics Gets Frothy as Mathematicians Dissect Mister Bubble.”
References
[1] K. Brakke, The Surface Evolver, Experiment. Math., 1:2 (1992), 141–165.
[2] R.I. Saye and J.A. Sethian, Analysis and applications of the Voronoi implicit interface method, J. Comput. Phys., 231:18 (2012), 6051–6085.
[3] R.I. Saye and J.A. Sethian, Multiscale modeling of membrane rearrangement, drainage, and rupture in evolving foams, Science, 340:6133 (2013), 720–724.
[4] R.I. Saye and J.A. Sethian, The Voronoi implicit interface method for computing multiphase physics, Proc. Natl. Acad. Sci. USA, 108:49 (2011), 19498–19503.
[5] D. Weaire, A fresh start for foam physics, Science, 349:6133 (2013), 693–694.
[6] D. Weaire and S. Hutzler, The Physics of Foam, Oxford University Press, Oxford, UK, 1999.
Robert Saye is an applied mathematician in the Mathematics Group of the Computational Research Division at the Lawrence Berkeley National Laboratory and also affiliated with the Department of Mathematics of the University of California at Berkeley. James Albert Sethian is a professor of mathematics at the University of California, Berkeley, and the head of the Mathematics Group at the United States Department of Energy's Lawrence Berkeley National Laboratory.