By Barry A. Cipra
Picture a hive of mathematical biologists, buzzing with excitement as they collect the sweet nectar of data and convert it into the geometric comb of understanding. Scouts zip off in a dozen directions, exploring the nooks and crannies of alternative new theories, then report back to the others, performing a PowerPoint waggle dance in hopes of conveying their enthusiasm for a possible new home. The hive, somehow making sense of all the competing reports, reaches consensus and, in a chaotic cloud, swarms off to a new nest.
Or picture a colony of computational biologists, emerging groggily from their underground network, milling about in apparent confusion until they get their code debugged, then marching ahead with quiet, serial determination until they fan out for the day’s foraging, leaving trails of numeric pheromones for each other to follow.
Or picture a plague of physicists descending on a field of biology. Solitary in nature, they stimulate one another into a gregarious horde, advancing in a broad front driven largely by fear that those coming up from behind will, if given the chance, eat them alive.
Picture all this, and you’ve got a fairly good idea of life at the workshop Insect Self-organization and Swarming, held in March 2011 at the Mathematical Biosciences Institute at Ohio State University. About a hundred researchers gathered to discuss the latest findings in a field that’s tailor-made for multidisciplinary research.
“This field is special and very effective because mathematicians and biologists actually rub shoulders and elbows together at the lab bench,” says Nigel Franks, an expert on ants at the University of Bristol. “It isn’t just a case of theoreticians sitting on high, sort of throwing theoretical proofs down at muddy-booted biologists. It’s a case of us really working together. And it’s just fabulous for that reason.”
Ants Do IT
Consider the notoriously industrious ant, say of the species Atta colombica, a Central and South American leaf cutter. Audrey Dussutour of the University of Toulouse described experiments she and colleagues have done to understand the “rules of the road” these invertebrates obey while scurrying between nest and foraging site. A. colombica travel in a more or less straight, pheromone-laced line, which means that, like commuters on a busy highway, they encounter two-way traffic.
Humans, of course, have adopted oft-violated traffic laws and rules of etiquette to accommodate their competing interests. Ants get by with something simpler, in part because they have a common goal: to get as much food to the nest as quickly as possible with a minimum of effort. A. colombica’s solution involves three lanes of travel. Outbound ants mainly take the center lane. Inbound leaf-laden ants also take the center lane, while inbound unladen ants tend to take the two outer lanes.
Other species also adhere to three lanes, although the rules governing who belongs in which lane can differ. Larger and laden ants tend to get the middle lane, most likely because they have greater inertia and less mobility: When two ants meet head on, the smaller, more nimble one is more likely to give way, veering either right or left. In a 2002 paper, Franks and Iain Couzin, now at Princeton, analyzed a mathematical model of formican collisions. The three-lane structure, they hypothesized, helps keep the trail well defined and straight.
Things are more interesting on narrow portions of the trail—a condition researchers can easily create in a laboratory setting. Dussutour and colleagues have compared ants’ solution of their traffic-flow problems on bridges 5 and 50 mm wide. Their findings suggest that simple, easily implemented rules can produce efficient, nearly optimal solutions. When an outbound A. colombica encounters an inbound leaf-laden ant along a bottleneck, it pulls over to the shoulder to let its less-maneuverable brother by. Inbound unladen ants, on the other hand, yield to their outbound counterparts. The exception occurs when an inbound unladen ant gets in line behind a laden ant, in which case it benefits from the laden ant’s right-of-way.
Indeed, this exception proves to be the rule: Even though they could easily pass the slower-moving laden ants, it’s common to see a string of unladen ants “drafting” on a leaf-bearing leader. Dussutour’s group found that the occurrence of large groups on wide bridges fell off at roughly the 3/5 power of group size, with no groups (in their experiments with thousands of ants) having more than 14 ants and the average group having only 1.9 ants. On narrow bridges, by contrast, the occurrence fell off at around the 1/5 power of group size, with 73 ants in the largest observed group and 5.2 in the average group. Moreover, inbound and outbound platoons tended to follow a strict alternation on the narrow bridge, while they proceeded more or less at random on the wider bridge. Ants, it seems, are good at taking turns, possibly because their close genetic kinship makes it advantageous to do so.
On occasion, an entire ant colony will up and move. In studying the factors that affect a colony’s decision making, Franks has found, for example, that house-hunting ants have a nifty way of estimating the size of a new nest. In essence they take a random walk within the site, depositing pheromone as they go and counting the number of times they encounter their own scent: The larger the number, the smaller the site. He has also found that no individual ant needs to investigate more than one new site for the colony as a whole to pick the best next site.
Franks has even experimented with forcing colonies to pick among equally desirable new homes. You might expect a colony to either freeze up or scatter, but almost invariably it comes to a quick, unanimous decision. (Franks has a way to speed up the process: destruction of the old nest. But even if he leaves it intact, ants are always on the move.) “Househunting ants have solved every problem we’ve thrown at them,” he says.
Bees Do IT
Bees are also expert househunters. Apiologists have long known that scouts keep careful track of direction and distance to potential new hive sites (as well as to better pollen sources) and report back by means of a “waggle dance.” Only more recently have they begun to tease out the details of how the hive decides among competing dances. In one case, at least, the short answer is vector arithmetic.
Take Apis mellifera, the European honeybee. A. mellifera nests in enclosed spaces, such as holes in trees—or boxes provided by researchers. Its “dream home,” according to honeybee expert Thomas Seeley of Cornell, is capacious (40 liters, say), high off the ground (5 meters), and with a small, invader-discouraging entrance (less than 15 square centimeters). Seeley and colleagues have done experiments in which they take a bunch of bees out to an isolated and somewhat inhospitable island and present them with a choice of several boxes for a new nest site, one of which is far superior to the others; the bees ultimately opt for the best site approximately 90% of the time.
Seeley likens the bees’ selection process to political campaigns; the scouts’ waggle dances are the equivalent of campaign ads. The greater a scout’s enthusiasm for a site, the longer she dances. During a campaign, which can go on for the better part of a day, dances advertising the weaker sites slowly fade away, like rallies for the weaker candidates who initially clutter presidential primaries.
One mechanism that speeds up consensus building is the “stop signal”: Bees actively discourage competing waggle dances by sidling up to the dancer and vigorously buzzing it. Although this signal had been heard for decades, its purpose was discerned only recently, thanks in part to directional speakers capable of mimicking it. The combination of honest enthusiasm and the negative feedback of stop signals, it seems, is enough for A. mellifera to make the best decision almost all the time.
For other species, there is no obvious “best” decision. Mary Myerscough, a mathematical biologist at the University of Sydney, described studies of swarming behavior in Apis florea, the red dwarf honeybee. A. florea is an open-nesting species: It typically builds nests that hang from tree branches. Because its requirements are less specific than those of its European cousin, the red dwarf honeybee can make do with settling on a general direction when the hive is ready to go. Myerscough and colleagues have found that the swarm takes off in what is, in effect, the vector sum of the directions indicated by the waggle dances.
However they reach the decision to depart, bees in flight make a parade of ants look orderly and linear. It’s virtually impossible to discern the direction of a swarm by following the trajectory of a random individual for a few seconds—each bee seems to be going nowhere in a hurry. Nonetheless, swarms manage to maintain cohesion over great distances. How do they do it?
An answer is beginning to emerge through an interplay of theory and observation. Using stationary cameras pointing up at the sky, researchers have filmed swarms in flight. What the cameras record is a mix of mostly slow-flying bees moving in more or less random directions, and a small subset of “streakers” headed in the direction of the overall swarm. The theory is that individual bees react to the motion of nearby bees, trying to match their neighbors’ trajectories while avoiding midair collisions, but that they respond especially to fast-moving neighbors. Presumably (and this remains to be demonstrated), the streakers are the scouts that originally chose the new nesting site. By streaking from the back to the front of the swarm and then more slowly returning from front to back, a scout could be keeping the swarm on track to the desired destination.
The model that Myerscough’s group has developed specifies that streakers (called “informed” bees) zip in a direction parallel to the line connecting the center of the swarm to the desired destination. (In the model, different informed bees may have different ideas as to exactly where they’re heading, again relying on the swarm to do the correct vector arithmetic.) They figure they’ve reached the front of the swarm when there are fewer than 10 bees within a preset threshold (20 cm in the model), at which point they fly more slowly in the opposite direction, until they find themselves again amidst fewer than 10 bees. Meanwhile, the model has the “uninformed” bees constantly adjust their flight paths by specifying each bee’s velocity at 10-msec intervals as a weighted combination of components corresponding to contributions of avoidance (don’t let anyone get too close), coherence (try to stay near the center of the swarm), alignment (watch the streakers), and randomness.
Simulations with 10 digital streakers guiding a swarm of 500 A. florea starting from a box 5 cm on a side and traveling for 20 seconds (2000 timesteps) show that the rules are capable of keeping a swarm together and heading in the right direction—dissent among the streakers slows the swarm’s progress but doesn’t prevent it. If all 10 streakers agree on a destination, the swarm motors along at approximately 1 m/sec (roughly a bee’s body length per timestep); even if 4 of the 10 want to go in the opposite direction, the swarm follows the majority, albeit with considerable dithering (see Figure 1). Only in the case of an exact 50:50 split—and in trials with no streakers—does the swarm fail to budge.
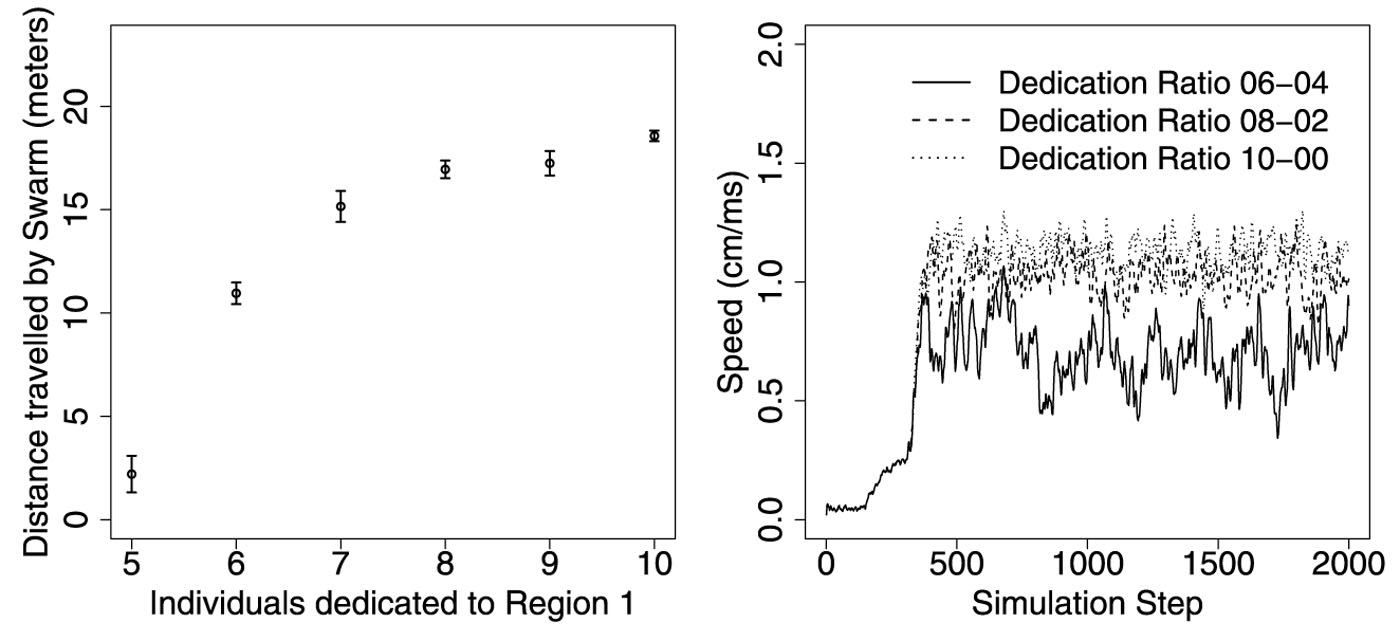
Figure 1. Pilgrims’ Progress. If k streakers are dedicated to a destination in Region 1 and 10-k streakers to a destination in the opposite direction, a simulated swarm of 500 bees makes progress in 2000 time steps (20 sec) unless there’s an exact 50:50 split (left), but the swarm’s average speed is lower and more variable if there is a lot of dissent (right). Figure courtesy of Konrad Diwold, Timothy M. Schaerf, Mary R. Myerscough, Martin Middendorf, and Madeleine Beekman: “Deciding on the wing: In-flight decision making and search space sampling in the red dwarf honeybee Apis florea,” Swarm Intelligence, 5:2 (2011),121-141.
|
Even Whirligigs Do IT
Ants and bees aren’t the only insects that exhibit orderly behavior in the midst of seemingly random motion. Locusts, such as Schistocerca gregaria, are well known for marshalling the repeated random jumps of individuals (interspersed with destructive munching) into an almost military march. One recent discovery, reported by Stephen Simpson of the University of Sydney, is how “shy, green, harmless grasshoppers” turn into brightly colored, voracious swarmers.
The trigger for the change, Simpson and colleagues found, is crowding. When S. gregaria individuals in their solitary phase bump into one another, touch-sensitive neurons in hairs on their back legs release a pulse of serotonin. In S. gregaria, the mood-altering neurotransmitter precipitates a radical, Jekyll-to-Hyde transformation. “We’ve got the soul of the locust, almost, in those two neurons,” Simpson says.
At some threshold of crowding, the locusts begin to move en masse. The global motion arises strictly from local interactions, although the insects have a somewhat grisly motivation to keep moving in one direction: The neurochemical transformation includes a sudden cannibalistic appetite for protein, and laggards are likely to wind up as so much locust lunch.
Whirligigs, as described by William Romey, a biologist at the State University of New York at Potsdam, form a somewhat more benign version of a gregarious group. A family (Gyrinidae) comprising some 700 species, whirligigs are tiny beetles that live on the surface of ponds and rivers; they are named for their peculiar spinning behavior. At night whirligigs tend to scatter and scavenge for food (mainly insects stuck in the surface tension of the water), but by day they clump together, primarily for safety’s sake—birds prey on them from above, fish from below.
Romey and colleagues have studied how whirligigs position themselves and circulate within a clump. (There is constant movement within a cluster, which itself usually wanders around the surface of the water.) Being on the perimeter has advantages, such as having first dibs on any food that floats by, as well as disadvantages: Predators inexplicably favor attacking at the margins. When predators and prey are constrained to the same dimensionality, such as lions attacking a herd of antelope, it makes sense for the predators to concentrate on the edges, which they’ll reach the earliest, but that’s not the case for predators that have an extra dimension to work with. Nonetheless, in a study published in 2007, Romey and colleagues A.R. Walston of Cornell and Penelope Watt of the University of Sheffield found that fish, specifically goldfish and bass, have a marked preference for the periphery of prey groups.
By manipulating the hunger level of whirligigs (and the demographics of sex and age), then watching how the beetles organize themselves when they circle the wagons, the researchers can test mathematical models that describe the group dynamics. The models indicate that simple rules can account for whirligigs’ meanderings. It’s possible, for example, to get one group of simulated beetles to linger on the perimeter while another group gravitates toward the center by using two different “attraction/repulsion” functions to describe the way a given beetle reacts to its nearest neighbors: Amped-up repulsion tends to drive an individual toward the edge. (It’s not clear that this drives actual whirligig swarms, but that’s one reason for running simulations. The models can be mined for quantitative predictions that suggest ex-periments on actual swarms.) In addition, models for evolutionary optimization indicate that the beetles’ group behavior is individually adaptive: It increases each beetle’s chance of survival, not just the group’s. Significantly, whirligigs—unlike bees and ants—are not closely related, so that altruism and kin selection are not high priorities.
The MBI workshop also featured talks on group dynamics of birds, fish, Princeton undergraduates, and robots. (The undergraduates were participants in a “flock logic” study conducted at Princeton by Naomi Leonard in collaboration with choreographer Susan Marshall. The basic idea was to record and analyze the motions of groups of “dancers” instructed, for example, to stay at arm’s length from at least two other dancers at all times while not actually bumping into anyone.) Overall, the mathematical study of insect and other swarms is revealing a rich range of phenomena that occur as emergent, self-organizing behavior. Someday it might even explain the traffic patterns around the coffee urns when a swarm of mathematicians take a break between talks.
Barry A. Cipra is a mathematician and writer based in Northfield, Minnesota.